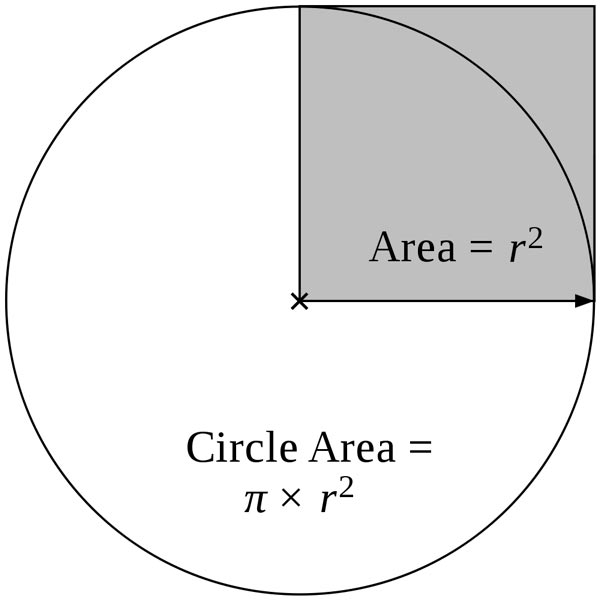
The below given is the area of segment of circle formula to calculate the area of circle segment on your own. As per the formula, deduct the value of θ by the value of sinθ and multiply the value by the squared value of radius. Then, divide the resultant value by the integer 2.
It might be the case that the chord that determines two segments of a circle is the diameter, in which case the segments will be semi-circles, which have area \frac . Anytime you cut a slice out of a pumpkin pie, a round birthday cake, or a circular pizza, you are removing a sector. A sector is created by the central angle formed with two radii, and it includes the area inside the circle from that center point to the circle itself. The portion of the circle's circumference bounded by the radii, the arc, is part of the sector. The segment of a circle definition and the concept of circular sector involve arcs of circumference and segments of the line. A circular sector is the region of a circle delimited by two radii and the arc of circumference subtended by them.
In Figure 1, for instance, the region that resembles a slice of pizza and is delimited by radii AC and AD and the arc \overset is a circular sector. The segment of a circle, the main topic of this lesson, will be addressed in the following section. Acute central angles will always produce minor arcs and small sectors.
When the central angle formed by the two radii is 90°, the sector is called a quadrant . When the two radii form a 180°, or half the circle, the sector is called a semicircle and has a major arc. A circle may be divided into two or more segments.
A segment is the region of a circle bounded by a chord and an arc. If the circle is divided into two segments, the bigger portion is called the major segment and the smaller portion is called the minor segment. The semi-circle is the biggest segment of any circle. A sector is the region bounded by two radii and an arc. A segment of a circle is a portion of the circle enclosed by a chord and an arc like a live of pizza.
Learn the formula used to calculate the area of a segment using examples with sectors and triangles. The Complete Circular Arc CalculatorSolves all twenty one cases when given any two inputs. This calculator calculates for the radius, length, width or chord, height or sagitta, apothem, angle, and area of an arc or circle segment given any two inputs. Please enter any two values and leave the values to be calculated blank.
There could be more than one solution to a given set of inputs. Please be guided by the angle subtended by the arc. If the angle is greater than 180 degrees then the arc length described is greater than the arc length of a semi-circle . The length unit choices are feet , inch , meter , centimeter , millimeter , yard , kilometer , mile .
Unlike triangles, the boundaries of sectors are not established by line segments. The distance along that curved "side" is the arc length. Pies, cakes, pizzas; so many foods we eat neatly lend themselves to mathematics, because they are models of circles. Bits cut off by connecting any two points on the circle are segments. Since both sectors and segments are part of a circle's interior, both have area. A segment of a circle is the part of a circle that is bounded by an arc of a circle and its chord.
Our online calculator calculates the area, arc length, chord length, height and perimeter of a circle segment. There are several options for calculating the segment parameters – by angle, by chord, by radius, by height and length of the arc.. The major segment consists of a major arc and the minor segment consists of a minor arc. Here it can be observed that ADC is the major segment and ABC is the minor segment. Besides, the area of these segments of a circle can also be calculated using formulas.
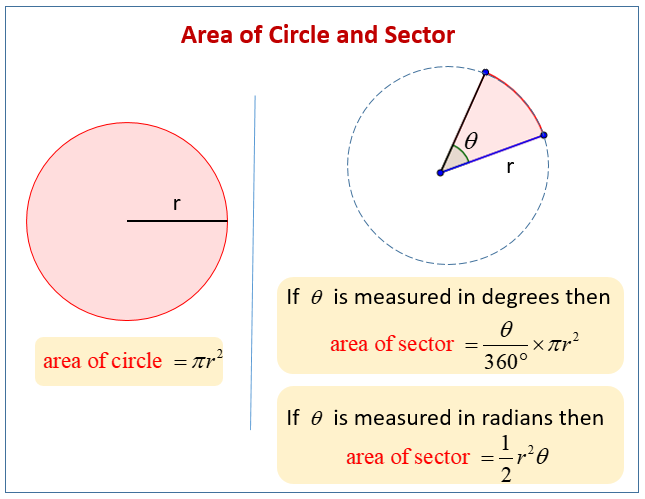
Angles are measured in degrees, but sometimes to make the mathematics simpler and elegant it's better to use radians which is another way of denoting an angle. A radian is the angle subtended by an arc of length equal to the radius of the circle. ( "Subtended" means produced by joining two lines from the end points of the arc to the center). In simple, a sector of a circle which is cut from the remaining portion of the circle by a secant or a chord can be said as the circular segment. Just substitute the values in the area of segment of circle formula and do the operations to get the results. There are two types of sectors, minor and major sector.
A minor sector is less than a semi-circle sector, whereas a major sector is a sector that is greater than a semi-circle. Theorem 1 Consider a circle, a chord, and a tangent to the circle through one of the endpoints of the chord. An alternate angle is an inscribed angle that subtends the given chord. The theorem states that the angle formed between the tangent and the chord is equal to the alternate angle. The following table gives the formulas for the area of sector and area of segment for angles in degrees or radians.
Scroll down the page for more explanations, examples and worksheets for the area of sectors and segments. A segment of a circle is the region that is bounded by an arc and a chord of the circle. There are two types of segments, one is a minor segment and the other is a major segment .
A portion of a disk whose upper boundary is a arc and whose lower boundary is a chordmaking a central angle radians (), illustrated above as the shaded region. The entire wedge-shaped area is known as a circular sector. If the chord is double the radius of a circle, the segments of a circle can be equal and referred to as semicircles.
If the angle between two radii is 180° the sectors can be referred to as semicircles. The measure of the central angle or the length of the arc. The central angle is the angle subtended by an arc of a sector at the center of a circle.
The central angle can be given in degrees or radians. A major segment is made by a major arc, and a minor arc makes a minor segment of the circle. Now, if you order pizza, how does it usually come? Those slices look like triangles with a curve, right?
A sector of a circle is the section enclosed by two radii and an arc . There are two types of segments, one is a minor segment, and the other is a major segment. A minor segment is made by a minor arc and a major segment is made by a major arc of the circle. One radian is equal to the angle formed when the arc opposite the angle is equal to the radius of the circle.
So in the above diagram, the angle ø is equal to one radian since the arc AB is the same length as the radius of the circle. Area of segment of a circle is obtained through subtracting the area of the triangle from the area of the sector. A line segment that joins two points on a circle or curve with both the end points lying on the arc is called a Chord. The region of the circle cut off from the rest of the circle by this chord is called as segment of a circle.
This segment is a part of a circle in two dimensional space bounded by a chord whose end points lie on the arc. In other words, the segments can be defined as the parts that are divided by the circle's arc and connected with the chord through its end points. Find the area of the circular segment if the diameter of a circle is 12 cm and the central angle is 4.59 radians. Using a pizza to help you understand the parts of a circle can be very helpful. Each semicircle has a diameter , radius, and chord, and each semicircle has an arc .
We divided the sector so that a triangle is formed and a segment is formed. If we were to consider the total area of that sector, we could say that the total area of the sector is made up of the area of the triangle and the area of the segment. However, their full slice includes the crust, plus the part that is left.
Therefore, we can say that a sector is made up of the triangle and the segment . Circular segment is a region of a circle which is "cut off" from the rest of the circle by a secant or a chord . Find the area of a segment of a circle, in which the radius of the circle it is in, is 6cm, and the central angle measures 120 degrees. The theorem states that in a circle, the angle which lies between the chord and tangent passing through the end points is equal to the angle in the alternate segment.
A segment of a circle can be described because of the place that's created with the aid of a secant or a chord with the corresponding arc of the circle. The section portraying a larger vicinity is known as the maximum critical section and the section having a smaller area is referred to as a minor segment. In step with the definition, a part of the round area that's enclosed between a chord and corresponding arc is known as a phase of the circle. There are classifications of segments in a circle, especially the critical section and the minor phase. The section that has a larger region is referred to as the essential phase and the section having a smaller region is referred to as the minor section.
A segment of a circle may be defined as an area bounded by using a chord and corresponding arc mendacity among the chord's endpoints. In exceptional terms, a round section is an area of a circle that is created by breaking aside from the rest of the circle through a secant or a chord. The following figure shows the major and minor segment of circle. One of the most common real-life examples of the area of a sector is a slice of a pizza.
The shape of slices of a pizza is similar to a sector of a circle. A pizza of \(7\) inches radius is divided into 6 equal-size slices, as shown in the below figure. Let us use the above logic to derive the formulas to find the segment of a circle both in degree and in radians.
As previously discussed, this is the area of the minor segment. The area of a segment of a circle can be broken down into major and minor . When you use the area of a segment of a circle formulas, you are calculating the minor segment area.
What Is The Formula For Circle Area To calculate the major area, you need to subtract the minor segment area away from the area of the circle. We are given radius of circle and angle that forms minor segment. In the figure below, the chord AB divides the circle into minor and major segments.
When a circle is divided into two segments of different areas, the biggest segment is called the major segment and the smaller segment is called the minor segment. Example 1 Evaluate the area of a minor segment of a circle whose radius is 3 cm and corresponding central angle is \frac . The area A of the circular segment is equal to the area of the circular sector minus the area of the triangular portion. When something is divided into parts, each part is referred to as a segment. In the same way, a segment is a part of the circle.
But a segment is not any random part of a circle, instead, it is a specific part of a circle that is cut by a chord of it. Let us learn about the definition of a segment of a circle and the formula to find the area of a segment of a circle in detail here. Usually, chord length and height are given or measured, and sometimes the arc length as part of the perimeter, and the unknowns are area and sometimes arc length. These can't be calculated simply from chord length and height, so two intermediate quantities, the radius and central angle are usually calculated first. A circular segment is enclosed between a secant/chord and the arc whose endpoints equal the chord's .
Measuring angles in radians enables us to write down quite simple formulas for the arc length of part of a circle and the area of a sector of a circle. The area of a sector of a circle is ½ r² ∅, where r is the radius and ∅ the angle in radians subtended by the arc at the centre of the circle. So in the below diagram, the shaded area is equal to ½ r² ∅ . Calculate the area of a segment of a circle with a central angle of 165 degrees and a radius of 4.
The segments of a circle can be classified into two types that are Major segment and Minor segment. The radius of a circle is 10 cm and the given angle is 30 degrees. Find the segment of the circle in radians using the area of segment formula. The region bounded by the chord is called the major segment and the major arc is intercepted by the chord. In short, the segment having a larger area is the major segment.
It has undeniably seeped into our day to day lives with its overwhelming versatility. The term circle finds its roots in the Greek word kirkos, which means hoop or circle. It also took influence from the Latin word circulus, which again means ring.